Singularity Functions
Singularity Functions are a class of functions that - you guessed it - contain singularities. One notation for a singularity function is given as adapted from p. 77 Chapra[1]
where \(x\)is the independent variable and \(n\) and \(x_0\) are constants. Note that this definition is only useful when \(n\geq 0\). Negative values of \(n\) are used to denote the impulse function (when \(n=-1\)) or its derivatives. Also note that it is common to leave the singularity undefined when \(n=0\) and \(x=x_0\). That is to say, a fuller definition of the singularity function might be:
where the delta function \(\delta(x)\) and its derivatives will be defined below.
Contents
Basic Definitions and Derivations
Alternate Names for \(n\geq 0\)
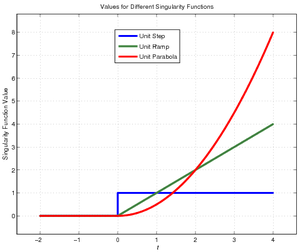
In courses such as ECE 54 and EGR 119, alternate names are given to different non-negative powers of the singularity function. Furthermore, singularity functions are generally written as functions of time rather than space, so:
For the last entry, you may be tempted to ask, "How is that the unit parabola?" The simplest explanation is that the unit ramp is the integral of the unit step, and the unit parabola is the integral of the unit parabola.
Derivation of Unit Impulse Functions
You can also take derivatives of the singularity functions. For \(n>0\), this is quite easy as the unit ramp and above are continuous. The difficulty comes in taking the derivative of the \(<t-a>^0\) case. Mathematically, call the derivative of the unit step function \(\delta(t)\); you can then find
But how large is that infinity? The answer comes in integrating this "delta function" (also known as the "unit impulse function" or just "impulse function"):
and by definition,
meaning
In other words (well...in words) the total area is 0 when integrating between negative infinity and just before 0 and the total area is 1 when integrating between negative infinity and anything positive. That must mean, at exactly \(t=0\), \(\delta(t)\) has an area of 1 while for all other times, it has an area of 0. That is to say,
Variable Transformations for Unit Step and Unit Impulse
Three common transformations of the independent variable (in the examples, \(t\)) are scaling, shifting, and reversing. The following pairs show how scaling (multiplying the independent variable by some positive constant \(a\)), shifting (subtracting some value \(t_0\) from the independent variable), and reversing (multiplying the independent variable by -1) may be written in alternate ways. First, for the unit step function:
Note that a positive time scaling does not have an effect on the unit step function. Also note in the final two cases above that the independent variable is first shifted, then scaled or scaled and reversed.
For the unit impulse function, time scaling is a bit more complex. Fortunately, time reversal is much simpler:
Convolution Integral Simplification with Step Function Product as Part of Integrand
The convolution integral
will generate products of step functions in the integrand if both \(x(t)\) and \(h(t)\) are defined using step functions. There are two main ways to get a product of step functions as an integrand - either they are both "pointing" in the same direction or they are pointing in opposite directions.
Same Directions in Integrand
If the step functions are "pointing" in the same direction in \(\tau\) space - that is
the key is to recognize that, for the integrand to be non-zero, you must satisfy the inequalities
You will thus need to determine which of these presents the stricter condition. Then:
which can be re-written using unit step functions as:
Opposite Directions in Integrand
This is the case that most often comes into play during convolution:
where the first step function is right-sided in \(\tau\) and the second function is left-sided. For the integrand to be nonzero,
This clearly indicates the limits on the integral - but it also indicates a restriction on time. That is, for both inequalities to be satisfied,
which gives rise to a unit step function that can be written outside the integral since it is not a function of \(\tau\). Specifically,
Questions
Post your questions by editing the discussion page of this article. Edit the page, then scroll to the bottom and add a question by putting in the characters *{{Q}}, followed by your question and finally your signature (with four tildes, i.e. ~~~~). Using the {{Q}} will automatically put the page in the category of pages with questions - other editors hoping to help out can then go to that category page to see where the questions are. See the page for Template:Q for details and examples.
External Links
References
- ↑ Applied Numerical Methods with MATLAB for Engineers and Scientists, 2/e, Steven C. Chapra